Project Description
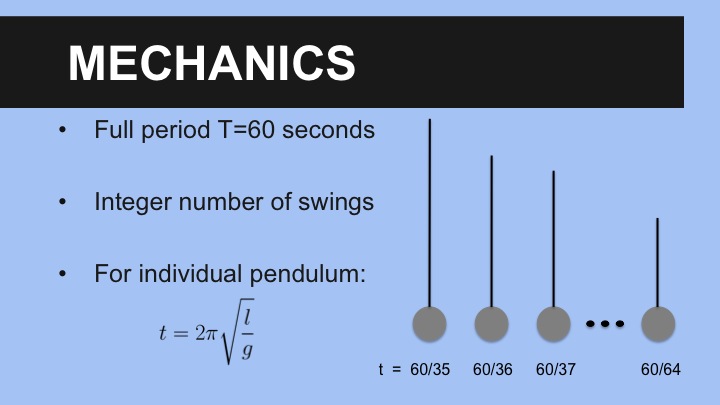
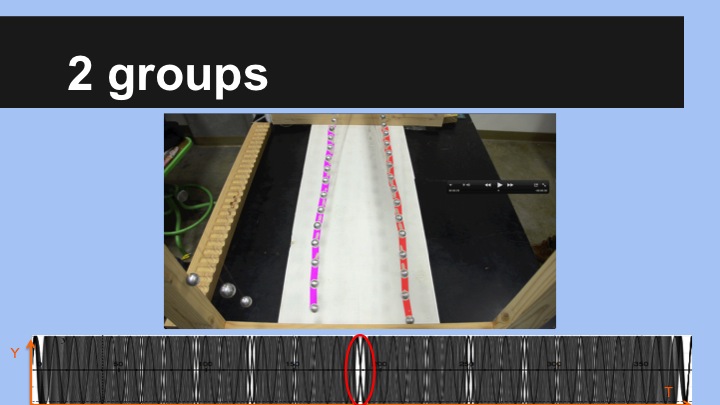
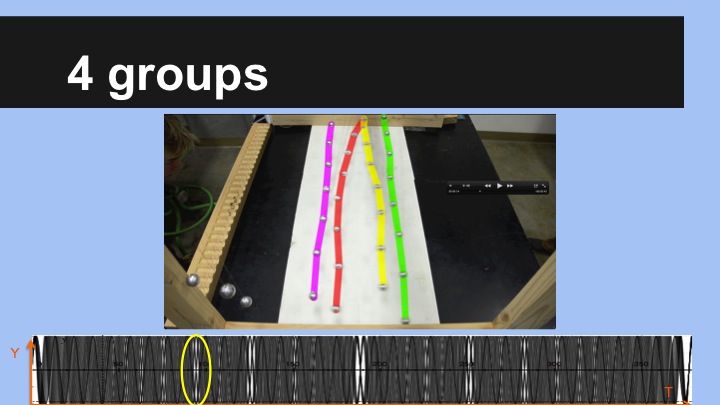
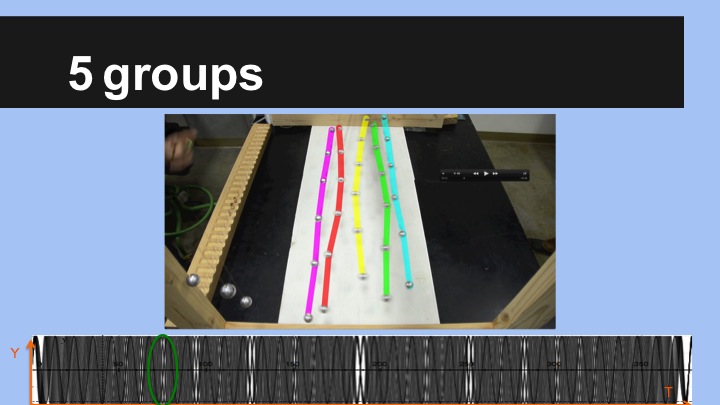
A harmonic pendulum is a series of uncoupled pendula with frequencies that change monotonically. Upon release from a uniform amplitude, the pendula display a variety of repeating patterns. We explored when and why these patterns happen by designing, building, and analyzing our own harmonic pendulum consisting of 30 independent swinging weights suspended by string of varying and specific lengths. We found that the phase differences between pendula cause distinct groups to form when the pendulum is a harmonic series fraction through its own period. For a harmonic pendulum with a period of 60 seconds, we found that n(n − 1) pendula guarantees the appearance of n separate groups. With our 30 pendula we were able to distinguish up to n = 6 groups. In our analysis, we considered aliasing and how it is manifested in the harmonic pendulum. We also investigated parallels between musical harmonics and the patterns created by the pendula.